Number Skills
Sorting- objects sharing a common attribute are grouped together.
One-to-one correspondence – this is necessary before can begin.
Counting- matching number names in one-to-one correspondence to a set of object.
Ordinality- ordering according to position
Cardinality- identifying that is the same number of members.
Conservation- recognizing that number is conserved whatever the arrangement of the members of the set.
Symbol recognition- recognising the abstract number symbol and relating them to the sound of the number words.
Place value- the position of a digit indicates its value, for example, 7, 73 and 741 each assign a different value to the digit 7.
Number operations
Addition- counting on from an identified point
Subtraction- taking away object from a set
- Working out the difference between 2 sets
Multiplication- adding equal sets (2+2+2=)
- enlargement (2,4,6,8,10)
- arrays e.g. 2 of bread = 6 types of
3 types of fixing sandwich
- sharing amounts equally between a given number of sets.
Division- establishing how many sets of a given number can be made
There are 6 fish
(e.g. how many cats can have 3 fish each? )
Fractional parts-
1/3 can represent:
- one whole divided into there parts
- a of a number that is divisible by three
- a share of a number less than three
- a share of a number not divisible by three
- a share of three
- a share of a number less than one
ALGEBRA- a generalisation of numerical relationships
Number patterns- portrayed numerically, algebraically and geometrically
e.g. patterns of number bonds
Patterns in place value
Patterns of multiplication and division
Patterns of equivalence and specific number patterns
Manipulation of unknown quantities.
- use of letters and symbols
- use of graphs and co-ordinates

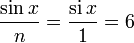
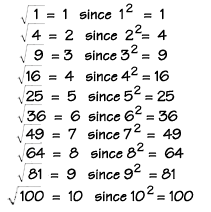
 In the rush to produce more and more crops to satisfy growing demand producers have had to resort to using a lethal cocktail of pesticides to control disease and insect attack.
In the rush to produce more and more crops to satisfy growing demand producers have had to resort to using a lethal cocktail of pesticides to control disease and insect attack.  Also don't forget about the cocktail of anti-biotics and hormones that cattle and poultry are force fed.
Also don't forget about the cocktail of anti-biotics and hormones that cattle and poultry are force fed.  Sure there are issues with availability and cost but with a bit of research you should be able to find local stores who stock organic produce.
Sure there are issues with availability and cost but with a bit of research you should be able to find local stores who stock organic produce. 
